2.2.2.3 Land and sea combined
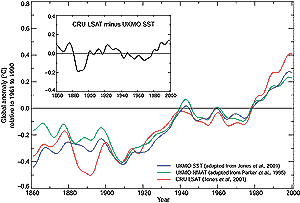
Figure 2.6: Smoothed annual anomalies of global average sea
surface temperature (°C) 1861 to 2000, relative to 1961 to 1990
(blue curve), night marine air temperature (green curve), and land-surface
air temperature (red curve). The data are from UK Met Office and
CRU analyses (adapted from Jones et al., 2001, and Parker et al.,
1995). The smoothed curves were created using a 21-point binomial
filter giving near-decadal averages. Also shown (inset) are the
smoothed differences between the land-surface air and sea surface
temperature anomalies. |
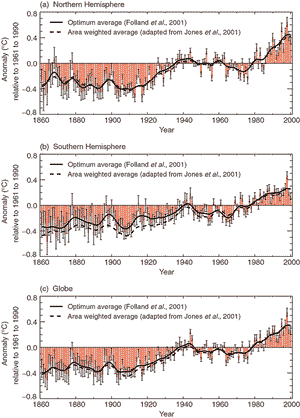
Figure 2.7: Smoothed annual anomalies of combined land-surface
air and sea surface temperatures (°C), 1861 to 2000, relative
to 1961 to 1990, for (a) Northern Hemisphere; (b) Southern Hemisphere;
and (c) Globe. The smoothed curves were created using a 21-point
binomial filter giving near-decadal averages. Optimally averaged
anomalies (Folland et al., 2001) – solid curves; standard area weighted
anomalies (adapted from Jones et al., 2001) – dashed curves. Also
shown are the unsmoothed optimum averages – red bars, and twice
their standard errors – width denoted by black “I”. Note that optimum
averages for the Southern Hemisphere are a little warmer before
1940, when the data are sparse, than the area-weighted averages.
However, the two types of averaging give similar results in the
Northern Hemisphere. |
|
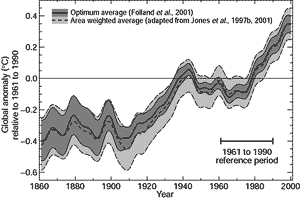
Figure 2.8: Smoothed annual anomalies of global combined land-surface
air and sea surface temperatures (°C), 1861 to 2000, relative to 1961
to 1990, and twice their standard errors. The smoothed curves and shaded
areas were created using a 21-point binomial filter giving near-decadal
averages, with appropriate errors. Optimally averaged anomalies and uncertainties
(Folland et al., 2001) – solid curve and dark shading; standard area
weighted anomalies and uncertainties (adapted from Jones et al., 1997b,
2001) – dashed curve and light shading. Note that uncertainties decrease
after 1941 due to the cessation of uncertainties due to bias corrections
in sea surface temperature. On the other hand, uncertainties due to urbanisation
of the land component, assessed as zero in 1900, continue to increase
after 1941 to a maximum in 2000.
|
Figure 2.6 summarises the relative
changes of UKMO SST, UKMO NMAT and CRU land-surface air temperature. The greater
warming of the land in recent years is clear, but otherwise all three curves
have a generally similar shape except that modest cooling of NMAT in the late
19th century is not seen in the SST data as noted for Figure
2.5. The relative coldness of the land around 1885 to 1895 comes from the
Northern Hemisphere continental interiors, particularly in winter, as global
coastal land air temperature and adjacent SST anomalies agree well at this time
(Parker et al., 1995), confirmed by the Jones et al. (2001) data. Note that
there are some systematic compensating differences between the land and SST
in the late 19th century in both hemispheres (not shown). The CRU land data
are generally about 0.1 to 0.2°C colder in the Northern Hemisphere except
at the beginning of the record (early 1860s), when they agree, and rather colder
than this in 1885 to 1890. The opposite is seen in the Southern Hemisphere before
1885 when SST is generally 0.1 to 0.2°C colder and 0.3°C colder around
1875. Overall the SST data are less variable in each hemisphere in these rather
poorly observed periods. The Southern Hemisphere land temperature at this time
can actually represent a very small observed area of the hemisphere while the
SST data, though sparse, are generally considerably more widespread. The sharp
cooling in SST around 1903/4 in Figures 2.5 and
2.6, seen in the land as well as the two ocean surface
data sets, was discussed for the North Atlantic and Indian Oceans by Helland-Hansen
and Nansen (1920) not long after the event. The reduced warming of the NMAT
in the last decade reflects differences in the Southern Hemisphere discussed
above. Slightly greater warming of the global ocean than the global land in
1910 to 1945 (seen in Table 2.1) is within the uncertainties
of either data set, as a slightly slower warming of the ocean might be expected
on physical grounds.
Figures 2.7a to c show annual time-series of anomalies
of combined land-surface air temperature and SST for the hemispheres and globe
since 1861, based on the latest CRU land air temperature data and the UKMO SST
data. Jones et al. (2001) temperature data have been averaged by both a standard
weighting method, used in the SAR, as shown by the dashed smoothed curves, and
by an optimum averaging method (Shen et al., 1998; Folland et al., 2001) as
shown by the bars and solid smoothed curves. The latter method uses the variance-covariance
matrix instead of correlation functions (Kagan, 1997). The calculated
uncertainties (twice the standard error) in the annual values are also shown
(including the independent urbanisation and SST bias correction uncertainties).
Optimum averaging gives less weight to areas of high data uncertainty than do
ordinary averaging methods, and it takes much better account of data gaps. It
also gives more weight to Antarctica, the great bulk of which (away from the
Antarctic Peninsula) has warmed little in the last two decades (Comiso, 2000).
Optimum averages can affect individual years markedly when data are sparse.
Thus extra warmth of the warm year 1878 (strongly affected by the 1877/78 El
Niño) in the Northern relative to the Southern Hemisphere in the area
weighted average (not shown) disappears when optimum averages are used. In the
Northern Hemisphere, the optimum averages are little different from area weighted
averages, but they are consistently warmer in the sparsely sampled Southern
Hemisphere before 1940, often by more than one tenth of a degree. The overall
effect on global temperature is small, however (Figure
2.7c)
The five warmest global optimally averaged years since the beginning of the
record in 1861 all occurred in the 1990s with 1998 having the warmest anomaly
(0.55°C). This year was significantly warmer than the second warmest year,
1995 (0.38°C), while 1999 was fourth warmest year, despite the strong La
Niña event. The remarkably consistent monthly global warmth of 1998 is
discussed in Karl et al. (2000).
Table 2.2 shows linear trends of the annual optimum
averages, and twice their standard errors, for the globe and hemispheres using
the restricted maximum likelihood method as in Table
2.1 and allowing for the annual uncertainties due to data gaps, urbanisation
over land, and bias corrections to SST. Since 1861 the hemispheres have warmed
by approximately the same amount. However both the earlier period of warming
(1910 to 1945) and the more recent one (1976 to 1999) saw rates of warming about
twice as great in the Northern Hemisphere. There was continued (non-significant)
warming in the Southern Hemisphere, though at a reduced rate, in 1946 to 1975,
which partially offset (non-significant) cooling in the Northern Hemisphere
over the same period to give a (non-significant) 0.03°C cooling globally.
The global trend from 1861 to 2000 can be cautiously interpreted as an equivalent
linear warming of 0.61°C over the 140-year period, with a 95% confidence
level uncertainty of ± 0.16°C. From 1901 an equivalent warming of
0.57°C has occurred, with an uncertainty of ± 0.17°C.
Figure 2.8 shows a smoothed optimally averaged
annual global time-series with estimates of uncertainty at ± twice the
standard error of the smoothed (near decadal) estimate. Note that the optimum
average uncertainties increase in earlier years mainly because of the much larger
data gaps. Also shown are uncertainties estimated by Jones et al. (1997b) using
a different method centred on the Jones et al. (2001) land and sea surface temperature
series. This series uses the average of anomalies from all available grid boxes,
weighted according to grid box area. Therefore, in contrast to the Jones et
al. (2001) global land-surface air temperature data, the global land and sea
surface temperature data are not a simple average of the hemispheres. The optimally
averaged uncertainties vary from about 15 to 65% less than those given by Jones
et al. (1997b). This is reasonable as optimum averages have minimum variance
amongst the range of unbiased estimates of the average. Not surprisingly, there
is relatively little difference in the decadal averages themselves. However
unlike the Jones et al. estimates of uncertainty, the optimum average also includes
uncertainties in bias corrections to SST up to 1941 (Folland and Parker, 1995)
and the uncertainties (as included in Figure 2.1)
in the land data component that are due to urbanisation. Cessation of the SST
component of uncertainty after 1941 is the reason for a lack of increase in
uncertainties in the fairly poorly observed period 1942 to 1945. Uncertainties
due to changes in thermometer screens are poorly known but could be 0.1°C
globally in the 19th and early 20th centuries (Parker, 1994); they are not included
here, but a preliminary analysis appears in Folland et al. (2001). For further
discussion of changes in land and ocean surface temperature, see Jones et al.
(1999a).
Referring back to Table 2.2 and including the second
decimal place, our best estimate of the equivalent linear rate of global land
and ocean surface warming between 1861 to 2000 is 0.044°C/decade, or a warming
of 0.61 ± 0.16°C. Over the period 1901 to 2000, the equivalent values
are 0.058°C/decade or a warming of 0.57 ± 0.17°C. These values
include the modifying effects of the annual uncertainties. So we calculate that
since the late 19th or the beginning of the 20th century, up to 2000, global
warming has been 0.6 ± 0.2°C. This is 0.15°C more warming than
the 0.3 to 0.6°C estimated more subjectively up to 1994 by the SAR. This
relatively large increase is explained by the increase in temperature since
the SAR was completed, improved methods of analysis and the fact that the SAR
decided not update the value in the First Assessment Report, despite slight
additional warming. The latter decision was likely to have been due to a cautious
interpretation of overall uncertainties which had at that time to be subjectively
assessed.
|